主题
Search

四边形

下载 Mathematica 笔记本下载 Wolfram 笔记本
Quadrilateral

四边形，有时也称为四角形或四边形 (Johnson 1929, p. 61) 是一个四边多边形。如果未明确声明，通常认为所有四个多边形顶点都位于一个平面内。（如果这些点不位于一个平面内，则该四边形称为斜四边形。）四边形有三种拓扑类型（Wenninger 1983, p. 50）：凸四边形（左图）、凹四边形（中图）和交叉四边形（或蝴蝶形，或领结形；右图）。

具有两边平行的四边形称为梯形，而具有对边平行的四边形称为平行四边形。

QuadrilateralVectors

对于平面凸四边形（上图左图），设边长为 a、b、c 和 d，半周长 s，以及多边形对角线 p 和 q。多边形对角线垂直当且仅当 a^2+c^2=b^2+d^2。

边长平方和的方程为

a^2+b^2+c^2+d^2=p^2+q^2+4x^2,
(1)

其中 x 是连接多边形对角线中点的线的长度（Casey 1888, p. 22）。

对于圆内接和外切四边形，外接圆和内切圆满足

2r^2(R^2-s^2)=(R^2-s^2)^2-4r^2s^2,
(2)

其中 R 是外接圆半径，r 是内切圆半径，s 是圆心距。

给定平面上处于一般位置的任意五个点，其中四个将构成一个凸四边形。这个结果是所谓的快乐结局问题的一个特例（Hoffman 1998, pp. 74-78）。

对于平面凸四边形的面积，有一个漂亮的公式，它用其两条对角线的向量表示。用向量 a、b、c 和 d 表示四边形的边，排列方式使得 a+b+c+d=0，并用向量 p 和 q 表示对角线，排列方式使得 p=b+c 和 q=a+b。那么

K=1/2|det(pq)|
(3)
=1/2|p×q|,
(4)

其中 det(A) 是行列式，pxq 是二维叉积。

对于平面凸四边形的面积，有许多漂亮的公式，它们用边长和对角线长度表示，包括

K=1/2pqsintheta
(5)
=1/4(b^2+d^2-a^2-c^2)tantheta
(6)

（Beyer 1987, p. 123），布雷特施奈德公式

K=1/4sqrt(4p^2q^2-(b^2+d^2-a^2-c^2)^2)
(7)
=sqrt((s-a)(s-b)(s-c)(s-d)-1/4(ac+bd+pq)(ac+bd-pq))
(8)

（Coolidge 1939；Ivanoff 1960；Beyer 1987, p. 123），其中 s 是半周长，以及漂亮的公式

K=sqrt((s-a)(s-b)(s-c)(s-d)-abcdcos^2[1/2(A+B)])
(9)

（Bretschneider 1842；Strehlke 1842；Coolidge 1939；Beyer 1987, p. 123）。

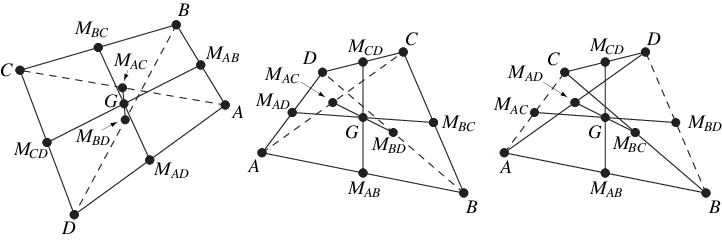
QuadrilateralCentroid

四边形顶点的质心位于双中线的交点（即，连接相对中点对的线 M_(AB)M_(CD) 和 M_(AD)M_(BC)）（Honsberger 1995, pp. 36-37）。此外，它也是连接对角线 AC 和 BD 的中点的线 M_(AC)M_(BD) 的中点（Honsberger 1995, pp. 39-40）。

QuadrilateralBisectors

四边形的四个角平分线在四个共圆点相交（Honsberger 1995, p. 35）。

QuadrilateralTiling

任何非自相交四边形都可以平铺平面。

四边形的四个点之间的六个距离 d_(12)、d_(13)、d_(14)、d_(23)、d_(24) 和 d_(34) 之间存在关系（Weinberg 1972）

0=d_(12)^4d_(34)^2+d_(13)^4d_(24)^2+d_(14)^4d_(23)^2+d_(23)^4d_(14)^2+d_(24)^4d_(13)^2+d_(34)^4d_(12)^2+d_(12)^2d_(23)^2d_(31)^2+d_(12)^2d_(24)^2d_(41)^2+d_(13)^2d_(34)^2d_(41)^2+d_(23)^2d_(34)^2d_(42)^2-d_(12)^2d_(23)^2d_(34)^2-d_(13)^2d_(32)^2d_(24)^2-d_(12)^2d_(24)^2d_(43)^2-d_(14)^2d_(42)^2d_(23)^2-d_(13)^2d_(34)^2d_(42)^2-d_(14)^2d_(43)^2d_(32)^2-d_(23)^2d_(31)^2d_(14)^2-d_(21)^2d_(13)^2d_(34)^2-d_(24)^2d_(41)^2d_(13)^2-d_(21)^2d_(14)^2d_(43)^2-d_(31)^2d_(12)^2d_(24)^2-d_(32)^2d_(21)^2d_(14)^2.
(10)

这可以通过将凯莱-门格尔行列式的左侧设置为

288V^2=|0 1 1 1 1; 1 0 d_(12)^2 d_(13)^2 d_(14)^2; 1 d_(21)^2 0 d_(23)^2 d_(24)^2; 1 d_(31)^2 d_(32)^2 0 d_(34)^2; 1 d_(41)^2 d_(42)^2 d_(43)^2 0|
(11)

等于 0（对应于体积为 0 的四面体）来最简单地推导出来，从而给出平面四边形的顶点之间的距离之间的关系（Uspensky 1948, p. 256）。

四边形的一种特殊类型是圆内接四边形，对于它，可以外接一个圆，使其与每个多边形顶点相切。另一种特殊类型是圆外切四边形，对于它，可以内切一个圆，使其与每条边相切。既是圆内接又是圆外切的四边形称为圆内接和外切四边形。

另请参阅

反心, 圆内接和外切四边形, 双中线, 婆罗摩笈多公式, 布雷特施奈德公式, 蝴蝶定理, 凯莱-门格尔行列式, 完全四边形, 圆内接四边形, 菱形, 八点圆定理, 等腰四边形, 法诺公理, 莱昂·安妮定理, 菱形, 高线, 垂心四边形, 平行四边形, 托勒密定理, 有理四边形, 矩形, 菱形, 斜四边形, 正方形, 圆外切四边形, 梯形, 范·奥贝尔定理, 瓦里尼翁定理, 维滕鲍尔平行四边形 在 MathWorld 课堂中探索此主题

使用 Wolfram|Alpha 探索

参考文献

Beyer, W. H. (Ed.). CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 123, 1987.Bretschneider, C. A. "Untersuchung der trigonometrischen Relationen des geradlinigen Viereckes." Archiv der Math. 2, 225-261, 1842.Casey, J. A Sequel to the First Six Books of the Elements of Euclid, Containing an Easy Introduction to Modern Geometry with Numerous Examples, 5th ed., rev. enl. Dublin: Hodges, Figgis, & Co., 1888.Coolidge, J. L. "A Historically Interesting Formula for the Area of a Quadrilateral." Amer. Math. Monthly 46, 345-347, 1939.Dostor, G. "Propriétés nouvelle du quadrilatère en général...." Archiv d. Math. u. Phys. 48, 245-348, 1868.Durell, C. V. "The Quadrilateral and Quadrangle." Ch. 7 in Modern Geometry: The Straight Line and Circle. London: Macmillan, pp. 77-87, 1928.Fukagawa, H. and Pedoe, D. "Circles and Quadrilaterals" and "Quadrilaterals." §3.5 and 4.2 in Japanese Temple Geometry Problems. Winnipeg, Manitoba, Canada: Charles Babbage Research Foundation, pp. 43-45, 47-48, and 125-132, 1989.Harris, J. W. and Stocker, H. "Quadrilaterals." §3.6 in Handbook of Mathematics and Computational Science. New York:Springer-Verlag, pp. 82-86, 1998.Hobson, E. W. A Treatise on Plane and Advanced Trigonometry. New York: Dover, pp. 204-205, 1957.Hoffman, P. The Man Who Loved Only Numbers: The Story of Paul Erdős and the Search for Mathematical Truth. New York: Hyperion, 1998.Honsberger, R. "On Quadrilaterals." Ch. 4 in Episodes in Nineteenth and Twentieth Century Euclidean Geometry. Washington, DC: Math. Assoc. Amer., pp. 35-41, 1995.Ivanoff, V. F. "Solution to Problem E1376: Bretschneider's Formula." Amer. Math. Monthly 67, 291-292, 1960.Johnson, R. A. "Quadrangles and Quadrilaterals" and "The Theorem of Ptolemy." §91-92 in Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 61-64, 1929.Routh, E. J. "Moment of Inertia of a Quadrilateral." Quart. J. Pure Appl. Math. 11, 109-110, 1871.Strehlke, F. "Zwei neue Sätze vom ebenen und shparischen Viereck und Umkehrung des Ptolemaischen Lehrsatzes." Archiv der Math. 2, 33-326, 1842.Uspensky, J. V. Theory of Equations. New York: McGraw-Hill, p. 256, 1948.Weinberg, S. Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity. New York: Wiley, p. 7, 1972.Wenninger, M. J. Dual Models. Cambridge, England: Cambridge University Press, 1983.

在 Wolfram|Alpha 上被引用

四边形

请引用为

Eric W. Weisstein "四边形。" 来自 MathWorld--Wolfram Web 资源。 https://mathworld.net.cn/Quadrilateral.html

主题分类