参见
反踏瓣曲线,
负踏瓣曲线,
踏瓣点
使用 Wolfram|Alpha 探索
参考文献
Ameseder, A. "Ueber Fusspunktcurven der Kegelschnitte." Archiv Math. u. Phys. 64, 143-144, 1879.Ameseder, A. "Zur Theorie der Fusspunktencurven der Kegelschnitte." Archiv Math. u. Phys. 64, 145-163, 1879.Gray, A. "Pedal Curves." §5.8 in Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, pp. 117-125, 1997.Hilbert, D. and Cohn-Vossen, S. Geometry and the Imagination. New York: Chelsea, p. 25, 1999.Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 46-49 and 204, 1972.Lockwood, E. H. "Pedal Curves." Ch. 18 in A Book of Curves. Cambridge, England: Cambridge University Press, pp. 152-155, 1967.Porteous, I. R. Geometric Differentiation for the Intelligence of Curves and Surfaces. Cambridge, England: Cambridge University Press, 1994.Trott, M. The Mathematica GuideBook for Graphics. New York: Springer-Verlag, p. 19, 2004. http://www.mathematicaguidebooks.org/.Ueda, K. In Mathematical Methods for Curves and Surfaces (Ed. T. Lyche and L. L. Shumaker). Nashville, TN: Vanderbilt University Press, 2001.Yates, R. C. "Pedal Curves." A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 160-165, 1952.Zwikker, C. The Advanced Geometry of Plane Curves and Their Applications. New York: Dover, pp. 150-158, 1963.在 Wolfram|Alpha 中被引用
踏瓣曲线
请引用为
韦斯坦因，埃里克·W. "踏瓣曲线。" 来自 MathWorld--Wolfram 网络资源。 https://mathworld.net.cn/PedalCurve.html
主题分类
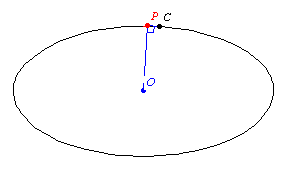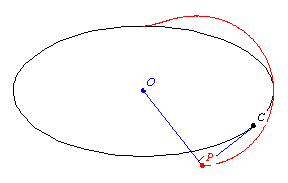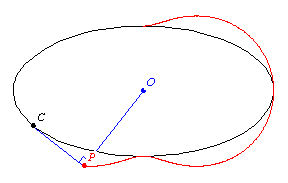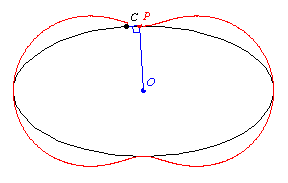
关于点 
的踏瓣曲线是从 
到曲线切线的垂足的轨迹。更准确地说，给定曲线 
，关于固定点 
（称为踏瓣点）的 
的踏瓣曲线 
是从 
到 
的切线的垂线的交点 
的轨迹。相对于踏瓣点 
，曲线 
的参数方程由下式给出
是曲线 
的踏瓣曲线，则 
是 
的负踏瓣曲线 (Lawrence 1972, pp. 47-48)。