主题
Search

正切

DOWNLOAD Mathematica Notebook下载 Wolfram 笔记本
Tangent

正切函数定义为

tanx=(sinx)/(cosx),
(1)

其中 sinx 是 正弦 函数，cosx 是 余弦 函数。符号 tgx 有时也使用（Gradshteyn 和 Ryzhik 2000，第 xxix 页）。

TangentDiagram

在 直角三角形 中，角度 theta 的正切的常见教科书定义（等同于刚刚给出的定义）是角对边边长与角邻边边长的比率，即

tantheta=(opposite)/(adjacent).
(2)

记住 正弦、余弦和正切定义的便捷助记符是 SOHCAHTOA （正弦 等于对边除以斜边，余弦 等于邻边除以斜边，正切等于对边除以邻边）。

“正切”一词也具有重要的相关含义，指在单个点接触给定曲线或实体的 直线 或 平面。这些几何对象然后分别称为 切线 或 切平面。

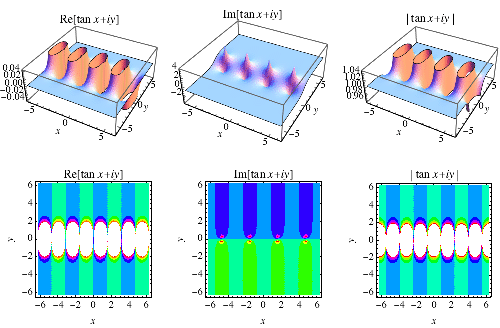
TanReImAbs
最小值 最大值
实部
虚部 Powered by webMathematica

正切函数的定义可以扩展到复数参数 z，使用定义

tanz=(i(e^(-iz)-e^(iz)))/(e^(-iz)+e^(iz))
(3)
=(e^(iz)-e^(-iz))/(i(e^(iz)+e^(-iz)))
(4)
=(i(1-e^(2iz)))/(1+e^(2iz))
(5)
=(e^(2iz)-1)/(i(e^(2iz)+1)),
(6)

其中 e 是 自然对数 的底数，i 是 虚数。正切在 Wolfram 语言 中实现为Tan[z]。

一个相关的函数，称为 双曲正切，类似地定义为，

tanhz=(e^z-e^(-z))/(e^z+e^(-z)).
(7)

一个重要的正切恒等式由下式给出

tan^2theta+1=sec^2theta.
(8)

角和、差、半角和倍角公式由下式给出

tan(alpha+beta)=(tanalpha+tanbeta)/(1-tanalphatanbeta)
(9)
tan(alpha-beta)=(tanalpha-tanbeta)/(1+tanalphatanbeta)
(10)
tan(2alpha)=(2tanalpha)/(1-tan^2alpha)
(11)
tan(nalpha)=(tan[(n-1)alpha]+tanalpha)/(1-tan[(n-1)alpha]tanalpha)
(12)
tan(alpha/2)=(sinalpha)/(1+cosalpha)
(13)
=(1-cosalpha)/(sinalpha)
(14)
=(tanalphasinalpha)/(tanalpha+sinalpha).
(15)

正弦 和 余弦 函数可以方便地用正切表示为

cost=(1-tan^2(1/2t))/(1+tan^2(1/2t))
(16)
sint=(2tan(1/2t))/(1+tan^2(1/2t)),
(17)

这在多项式计算中可能特别方便，例如 Gröbner 基，因为它减少了方程的数量，与显式包含 cost 和 sint 以及附加关系 cos^2t+sin^2t-1=0 相比（Trott 2006，第 39 页）。

这些导致了漂亮的恒等式

tan(x+1/4pi)=(1+tanx)/(1-tanx).
(18)

对于三个变量，还有一个美丽的角和恒等式，

tan(alpha+beta+gamma)=(tanalpha+tanbeta+tangamma-tanalphatanbetatangamma)/(1-tanbetatangamma-tangammatanalpha-tanalphatanbeta).
(19)

另一个正切恒等式是

tan(nx)=(sum_(k=0)^(n)(-1)^k(n; 2k+1)t^(2k+1))/(sum_(k=0)^(n)(-1)^k(n; 2k)t^(2k))
(20)
=(1/2i(1-it)^n-(1+it)^n)/(1/2(1-it)^n+(1+it)^n)
(21)
=1/i((1+it)^n-(1-it)^n)/((1+it)^n+(1-it)^n),
(22)

其中 t=tanx （Beeler et al. 1972）。显式写出，

tan(nx)=(2i(1-itant)^n)/((1-itant)^n+(1+itant)^n)-i,
(23)

这给出了前几个展开式为

tanx=t
(24)
tan(2x)=(2t)/(1-t^2)
(25)
tan(3x)=(3t-t^3)/(1-3t^2)
(26)
tan(4x)=(4t-4t^3)/(1-6t^2+r^4)
(27)
tan(5x)=(5t-10t^3+t^5)/(1-10t^2+5t^4)
(28)

（OEIS A034867 和 A034839）。

一个推广正切角和公式的漂亮公式，（27）和（28）由下式给出

tan(sum_(n=1)^Ntheta_n)=i(product_(n=1)^(N)(1-itantheta_n)-product_(n=1)^(N)(itantheta_n+1))/(product_(n=1)^(N)(itantheta_n+1)+product_(n=1)^(N)(1-itantheta_n))
(29)

（Szmulowicz 2005）。

有许多基于上面给出的简单但有趣的正切恒等式，包括

tan(A+60 degrees)tan(A-60 degrees)+tanAtan(A+60 degrees)+tanAtan(A-60 degrees)=-3
(30)

（Borchardt 和 Perrott 1930）。

对于 -pi/2<x<pi/2 有效的 麦克劳林级数 对于正切函数是

tanx=sum_(n=1)^(infty)((-1)^(n-1)2^(2n)(2^(2n)-1)B_(2n))/((2n)!)x^(2n-1)
(31)
=x+1/3x^3+2/(15)x^5+(17)/(315)x^7+(62)/(2835)x^9+...
(32)

（OEIS A002430 和 A036279），其中 B_n 是 伯努利数。

对于任何 有理数 x!=0，tanx 是 无理数，这可以通过将 tanx 写成 连分数 来证明，如

tanx=x/(1-(x^2)/(3-(x^2)/(5-(x^2)/(7-...))))
(33)

（Wall 1948，第 349 页；Olds 1963，第 138 页）和

tanx=1/(1/x-1/(3/x-1/(5/x-1/(7/x-...)))).
(34)

两者都归功于 Lambert。

一个有趣的涉及正切 乘积 的恒等式是

product_(k=1)^(|_(n-1)/2_|)tan((kpi)/n)={sqrt(n) for n odd; 1 for n even,
(35)

其中 |_x_| 是 向下取整函数。

方程

x=tanx,
(36)

它等价于 tanc(x)=1，其中 tanc(x) 是 tanc 函数，没有简单的闭式解。

对于更高阶的解，连续解之间的差异越来越接近 pi。函数 tancx=(tanx)/x 有时被称为 tanc 函数。

另请参阅

交错排列、余弦、余切、双曲正切、反切、正切定理、莫里定律、正弦、SOHCAHTOA、Tanc 函数、切线、切平面、切向量、瓦尔迪积分 在 MathWorld 课堂中探索此主题

相关 Wolfram 网站

http://functions.wolfram.com/ElementaryFunctions/Tan/

使用 Wolfram|Alpha 探索

参考文献

Abramowitz, M. 和 Stegun, I. A. (Eds.). "Circular Functions." §4.3 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 71-79, 1972.Beeler, M. et al. Item 16 in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, p. 9, Feb. 1972. http://www.inwap.com/pdp10/hbaker/hakmem/recurrence.html#item16.Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 226, 1987.Borchardt, W. G. 和 Perrott, A. D. Ex. 33 in A New Trigonometry for Schools. London: G. Bell, 1930.Gradshteyn, I. S. 和 Ryzhik, I. M. Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, 2000.Jeffrey, A. "Trigonometric Identities." §2.4 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 111-117, 2000.Olds, C. D. Continued Fractions. New York: Random House, 1963.Sloane, N. J. A. Sequences A002430/M2100, A034839, A034867, A036279, and A115365 in "The On-Line Encyclopedia of Integer Sequences."Szmulowicz, F. "New Analytic and Computational Formalism for the Band Structure of N-Layer Photonic Crystals." Phys. Lett. A 345, 469-477, 2005.Spanier, J. 和 Oldham, K. B. "The Tangent tan(x) and Cotangent cot(x) Functions." Ch. 34 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 319-330, 1987.Tropfke, J. Teil IB, §2. "Die Begriffe von Tangens und Kotangens eines Winkels." In Geschichte der Elementar-Mathematik in systematischer Darstellung mit besonderer Berücksichtigung der Fachwörter, fünfter Band, zweite aufl. Berlin and Leipzig, Germany: de Gruyter, pp. 23-28, 1923.Trott, M. The Mathematica GuideBook for Symbolics. New York: Springer-Verlag, 2006. http://www.mathematicaguidebooks.org/.Wall, H. S. Analytic Theory of Continued Fractions. New York: Chelsea, 1948.Zwillinger, D. (Ed.). "Trigonometric or Circular Functions." §6.1 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 452-460, 1995.

在 Wolfram|Alpha 上被引用

正切

请引用为

Weisstein, Eric W. "正切。" 来源 MathWorld--Wolfram Web 资源。 https://mathworld.net.cn/Tangent.html

学科分类