第一 Brocard 点 是三角形  的内部点
的内部点  (也记作
(也记作  或
或  ),其中点以逆时针顺序标记,使得角
),其中点以逆时针顺序标记,使得角  、
、 和
和  相等,且唯一的这样的角记为
相等,且唯一的这样的角记为  。它不是一个三角形中心,但具有三线坐标
。它不是一个三角形中心,但具有三线坐标
 |
(1)
|
(Kimberling 1998, p. 47)。
请注意,在查阅文献时需要格外小心,因为反转三角形顶点的标记顺序会导致 Brocard 点的交换。
第二 Brocard 点 是内部点  (也记作
(也记作  或
或  ),使得角
),使得角  、
、 和
和  相等,且唯一的这样的角记为
相等,且唯一的这样的角记为  。它不是一个三角形中心,但具有三线坐标
。它不是一个三角形中心,但具有三线坐标
 |
(2)
|
(Kimberling 1998, p. 47)。
此外,两个角  相等,并且这个角被称为Brocard 角,
相等,并且这个角被称为Brocard 角,
前两个 Brocard 点是等角共轭点(Johnson 1929, p. 266)。它们由法国陆军军官 Henri Brocard 于 1875 年描述,尽管 Jacobi 曾在此之前进行过研究,而 Crelle 在 1816 年也研究过(Wells 1991;Honsberger 1995, p. 98)。它们满足
 |
(5)
|
其中  是外心,
是外心, 是外接圆半径,并且
是外接圆半径,并且  ,其中
,其中  是外心,
是外心, 是Brocard 角(Honsberger 1995, p. 106)。
是Brocard 角(Honsberger 1995, p. 106)。
通常的说法(Bernhart 1959;Wells 1991, pp. 21-22;Marshall et al. 2005)归因于 Brocard,以回应 Edouard Lucas 在 1877 年提出的问题,即如果三只狗从三角形的顶点出发,并以恒定速度追逐它们的左邻或右邻,则三只狗将在  或
或  处相遇,这是不正确的。通过考虑一个接近共线的等腰三角形 可以看出这一点,并注意到其中两只狗需要比另一只狗走得远得多,因此不可能以相同的速度行进(参见 Peterson 2001,Nester),以便在点
处相遇,这是不正确的。通过考虑一个接近共线的等腰三角形 可以看出这一点,并注意到其中两只狗需要比另一只狗走得远得多,因此不可能以相同的速度行进(参见 Peterson 2001,Nester),以便在点  或
或  之一处与其他两只狗相遇。
之一处与其他两只狗相遇。
一条 Brocard 线、一条三角形中线 和一条交心线(每种线中取一条)是共点的,其中  、
、 和
和  交于一点,其中
交于一点,其中  是三角形重心,
是三角形重心, 是交心点。类似地,
是交心点。类似地, 、
、 和
和  交于一点,该点是第一个点的等角共轭点(Johnson 1929, pp. 268-269;Honsberger 1995, pp. 121-124)。
交于一点,该点是第一个点的等角共轭点(Johnson 1929, pp. 268-269;Honsberger 1995, pp. 121-124)。
设  是通过顶点
是通过顶点  和
和  并与直线
并与直线  在
在  处相切的圆,对于
处相切的圆,对于  和
和  也是如此。那么圆
也是如此。那么圆  、
、 和
和  在第一 Brocard 点
在第一 Brocard 点  处相交。类似地,设
处相交。类似地,设  是通过顶点
是通过顶点  和
和  并与直线
并与直线  在
在  处相切的圆,对于
处相切的圆,对于  和
和  也是如此。那么圆
也是如此。那么圆  、
、 和
和  在第二 Brocard 点
在第二 Brocard 点  处相交(Johnson 1929, pp. 264-265;Honsberger 1995, pp. 99-100)。
处相交(Johnson 1929, pp. 264-265;Honsberger 1995, pp. 99-100)。
垂足三角形  和
和  是全等的,并且与三角形
是全等的,并且与三角形  相似(Johnson 1929, p. 269)。涉及 Brocard 点的长度包括
相似(Johnson 1929, p. 269)。涉及 Brocard 点的长度包括
 |
(6)
|
 |
(7)
|
将线段  、
、 和
和  延伸到
延伸到  的外接圆以形成
的外接圆以形成  ,并将线段
,并将线段  、
、 和
和  延伸以形成
延伸以形成  。那么
。那么  和
和  与
与  全等(Honsberger 1995, pp. 104-106)。
全等(Honsberger 1995, pp. 104-106)。
第三 Brocard 点 通过三角形中心函数与给定的三角形相关
 |
(8)
|
(Casey 1893, Kimberling 1994),并且是Kimberling 中心  。第三 Brocard 点
。第三 Brocard 点  (或
(或  或
或  )与Spieker 中心以及其三角形的内心的等张共轭点共线。
)与Spieker 中心以及其三角形的内心的等张共轭点共线。
另请参阅
Brocard 角、
Brocard 中点、
Brocard 三角形、
等 Brocard 中心、
第一 Brocard 点、
老鼠问题、
追逐曲线、
第二 Brocard 点、
第三 Brocard 点、
Yff 点
使用 探索
参考文献
Bernhart, A. "Polygons of Pursuit." Scripta Math. 24, 23-50, 1959.Casey, J. A Treatise on the Analytical Geometry of the Point, Line, Circle, and Conic Sections, Containing an Account of Its Most Recent Extensions, with Numerous Examples, 2nd ed., rev. enl. Dublin: Hodges, Figgis, & Co., p. 66, 1893.Coolidge, J. L. "The Brocard Figures." §1.5 in A Treatise on the Geometry of the Circle and Sphere. New York: Chelsea, pp. 60-84, 1971.Emmerich, A. Die Brocardschen Gebilde und ihre Beziehungen zu den verwandten merkwürdigen Punkten und Kreisen des Dreiecks. Berlin: Reimer, 1891.Gallatly, W. "The Brocard Points." §130 in The Modern Geometry of the Triangle, 2nd ed. London: Hodgson, p. 94, 1913.Honsberger, R. "The Brocard Points." Ch. 10 in Episodes in Nineteenth and Twentieth Century Euclidean Geometry. Washington, DC: Math. Assoc. Amer., pp. 98-124, 1995.Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 263-286, 1929.Kimberling, C. "Central Points and Central Lines in the Plane of a Triangle." Math. Mag. 67, 163-187, 1994.Kimberling, C. "Triangle Centers and Central Triangles." Congr. Numer. 129, 1-295, 1998.Kimberling, C. "Encyclopedia of Triangle Centers: X(76)=3rd Brocard Point." http://faculty.evansville.edu/ck6/encyclopedia/ETC.html#X76.Lachlan, R. An Elementary Treatise on Modern Pure Geometry. London: Macmillian, pp. 65-66 and 79-80, 1893.Lemoine, É. "Propriétés relatives a deux points  ,
,  du plan d'un triangle
du plan d'un triangle  qui se déduisent d'un point
qui se déduisent d'un point  quelconque di plan comme les points de Brocard de déduisent du point de Lemoine." Mathesis 6, Suppl. 3, 1-22, 1886.Marshall, J. A.; Broucke, M. E.; and Francis, B. A. "Pursuit Formations of Unicycles." Automata 41, 3-12, 2005. http://www.control.toronto.edu/~marshall/docs/MarBroFra-Auto-4141-final.pdf.Nester, D. "Mathematics Seminar: Beetle Centers of Triangles." http://www.bluffton.edu/mat/dept/seminar_docs/BeetleCenters/.PandD Software. "De punten van Brocard." http://www.pandd.demon.nl/brocard.htm.Peterson, I. "MathTrek: Pursuing Pursuit Curves." Jul. 16, 2001. http://www.maa.org/mathland/mathtrek_7_16_01.html.Stroeker, R. J. "Brocard Points, Circulant Matrices, and Descartes' Folium." Math. Mag. 61, 172-187, 1988.Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, pp. 21-22, 1991.
quelconque di plan comme les points de Brocard de déduisent du point de Lemoine." Mathesis 6, Suppl. 3, 1-22, 1886.Marshall, J. A.; Broucke, M. E.; and Francis, B. A. "Pursuit Formations of Unicycles." Automata 41, 3-12, 2005. http://www.control.toronto.edu/~marshall/docs/MarBroFra-Auto-4141-final.pdf.Nester, D. "Mathematics Seminar: Beetle Centers of Triangles." http://www.bluffton.edu/mat/dept/seminar_docs/BeetleCenters/.PandD Software. "De punten van Brocard." http://www.pandd.demon.nl/brocard.htm.Peterson, I. "MathTrek: Pursuing Pursuit Curves." Jul. 16, 2001. http://www.maa.org/mathland/mathtrek_7_16_01.html.Stroeker, R. J. "Brocard Points, Circulant Matrices, and Descartes' Folium." Math. Mag. 61, 172-187, 1988.Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, pp. 21-22, 1991.在 上被引用
Brocard 点
请引用为
Weisstein, Eric W. "Brocard 点。" 来自 Web 资源。 https://mathworld.net.cn/BrocardPoints.html
主题分类
的内部点
(也记作
或
),其中点以逆时针顺序标记,使得角
、
和
相等,且唯一的这样的角记为
。它不是一个三角形中心,但具有三线坐标
(也记作
或
),使得角
、
和
相等,且唯一的这样的角记为
。它不是一个三角形中心,但具有三线坐标
相等,并且这个角被称为Brocard 角,

是外心,
是外接圆半径,并且
,其中
是外心,
是Brocard 角(Honsberger 1995, p. 106)。
或
处相遇,这是不正确的。通过考虑一个接近共线的等腰三角形 可以看出这一点,并注意到其中两只狗需要比另一只狗走得远得多,因此不可能以相同的速度行进(参见 Peterson 2001,Nester),以便在点
或
之一处与其他两只狗相遇。
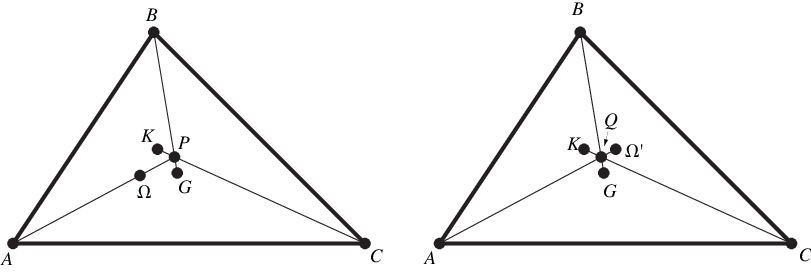
、
和
交于一点,其中
是三角形重心,
是交心点。类似地,
、
和
交于一点,该点是第一个点的等角共轭点(Johnson 1929, pp. 268-269;Honsberger 1995, pp. 121-124)。
是通过顶点
和
并与直线
在
处相切的圆,对于
和
也是如此。那么圆
、
和
在第一 Brocard 点
处相交。类似地,设
是通过顶点
和
并与直线
在
处相切的圆,对于
和
也是如此。那么圆
、
和
在第二 Brocard 点
处相交(Johnson 1929, pp. 264-265;Honsberger 1995, pp. 99-100)。
和
是全等的,并且与三角形
相似(Johnson 1929, p. 269)。涉及 Brocard 点的长度包括
、
和
延伸到
的外接圆以形成
,并将线段
、
和
延伸以形成
。那么
和
与
全等(Honsberger 1995, pp. 104-106)。
。第三 Brocard 点
(或
或
)与Spieker 中心以及其三角形的内心的等张共轭点共线。