可以构造出许多 привлекательных 十复合四面体。第一个(左图)可以通过组合具有相反手性的五复合四面体获得。
第一个十复合四面体的顶点位于十二面体-二十面体复合体和大星形十二面体的顶点之中。
上面图示的十复合四面体在 Wolfram 语言 中实现为PolyhedronData["TetrahedronTenCompound", n
] 对于
和 2。
这些十复合四面体与它们的对偶和共同的中球面一起在上面进行了图示。
共同的实体和凸包在上面进行了图示。对于第一个复合体,内部是正二十面体,凸包是正十二面体。对于第二个复合体,内部是平方-增广的立方八面体,凸包是倒角立方体。
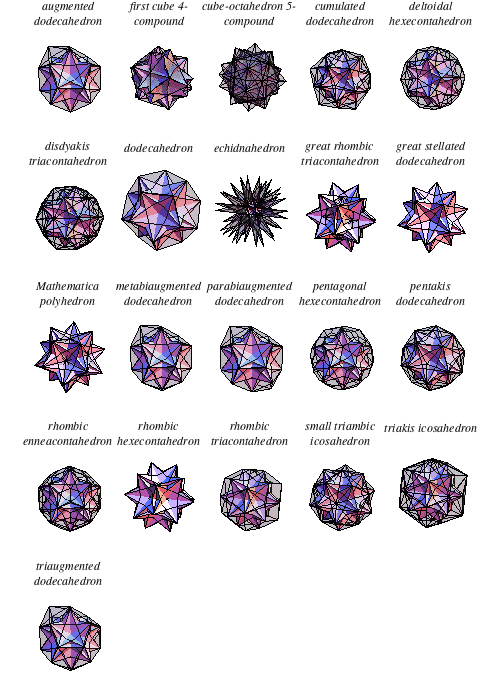
十复合四面体可以内接于增广十二面体,(第一个)四复合立方体,立方体-八面体五复合体,增广十二面体,三角六十面体,双三角三十面体,十二面体,棘刺二十四面体,大菱形三十面体,大星形十二面体,元增广十二面体,邻增广十二面体,五边形六十面体,五角十二面体,菱形九十面体,菱形六十面体,菱形三十面体,小三角三面体二十面体,尖刺状多面体,三面体二十面体,和三增广十二面体的顶点中(E. Weisstein,2009 年 12 月 25-28 日)。