向下取整函数

向下取整函数 , 也被称为最大整数函数或整数值 (Spanier and Oldham 1987), 给出小于或等于 的最大整数。向下取整函数的名称和符号由 K. E. Iverson (Graham et al. 1994) 创造。

不幸的是，在许多较旧和当前的作品中 (例如，Honsberger 1976, p. 30; Steinhaus 1999, p. 300; Shanks 1993; Ribenboim 1996; Hilbert and Cohn-Vossen 1999, p. 38; Hardy 1999, p. 18)，符号 被用来代替 (Graham et al. 1994, p. 67)。 事实上，这种表示法可以追溯到高斯在 1808 年对二次互反律的第三次证明。然而，由于向下取整函数和向上取整函数符号 和 的优雅对称性，并且由于 在被解释为 Iverson 括号时是一个非常有用的符号，因此应该弃用使用 来表示向下取整函数。 在这项工作中，符号 被用来表示最接近的整数函数，因为它自然地落在 和 符号之间。

最小值 最大值 实部 虚部

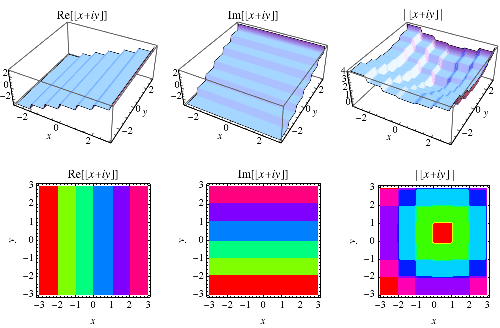
向下取整函数在 Wolfram 语言中实现为Floor[z]，其中它被推广到复数值 ，如上所示。

由于关于小数部分/值和整数部分/值的用法可能会令人困惑，下表总结了使用的名称和符号。这里，S&O 表示 Spanier 和 Oldham (1987)。

符号	名称	S&O	Graham 等人	Wolfram 语言
	向上取整函数	--	向上取整，最小整数	Ceiling[x]
	同余	--	--	Mod[m, n]
	向下取整函数		向下取整，最大整数，整数部分	Floor[x]
	小数数值		小数部分或	SawtoothWave[x]
	小数部分		无名称	FractionalPart[x]
	整数部分		无名称	IntegerPart[x]
	最接近的整数函数	--	--	Round[x]
	商	--	--	Quotient[m, n]

向下取整函数满足以下恒等式

(1)

对于所有整数 。

许多分子中带有向下取整函数的类几何序列可以进行解析计算。例如，以下形式的和

(2)

对于有理数 可以进行解析计算。对于 单位分数，

(3)

这种形式的和导致类似魔鬼阶梯的行为。

对于无理数 ，连分数收敛 ，以及 ,

(4)

（Borwein et al. 2004, p. 12）。这引出了一个相当惊人的结果，将 alpha 倍数的向下取整函数之和与 alpha 的连分数联系起来，通过

(5)

（Mahler 1929；Borwein et al. 2004, p. 12）。