主题
Search

几何质心

质心是二维平面薄片或三维实体的质量中心。具有表面密度函数 sigma(x,y) 的薄片的质量是

M=intintsigma(x,y)dA,
(1)

质心的坐标（也称为重心）是

x^_=(intintxsigma(x,y)dA)/M
(2)
y^_=(intintysigma(x,y)dA)/M.
(3)

薄片的质心是当将其放置在针尖上时能够保持平衡的点。固体的质心是固体能够“平衡”的点。

可以使用 Wolfram 语言 计算区域的几何质心，使用RegionCentroid[reg]。

一组 n 个点质量 m_i 位于位置 x_i 的质心是

x^_=(sum_(i=1)^(n)m_ix_i)/(sum_(i=1)^(n)m_i),
(4)

如果所有质量都相等，则简化为

x^_=(sum_(i=1)^(n)x_i)/n.
(5)

对于均匀密度的封闭薄片，其边界由 (x(t),y(t)) 指定，对于 t in [t_0,t_1] 且薄片位于曲线被遍历时的左侧，可以使用 格林定理 计算质心，如下所示

x^_=-1/(2A)int_(t_0)^(t_1)x^2y^'dt
(6)
y^_=1/(2A)int_(t_0)^(t_1)y^2x^'dt.
(7)

平面非自相交多边形的几何质心的位置，其顶点为 (x_1,y_1), ..., (x_n,y_n) 是

x^_=1/(6A)sum_(i=1)^(n)(x_i+x_(i+1))(x_iy_(i+1)-x_(i+1)y_i)
(8)
y^_=1/(6A)sum_(i=1)^(n)(y_i+y_(i+1))(x_iy_(i+1)-x_(i+1)y_i),
(9)

其中 A 是多边形面积，x_(n+1)=x_1 和 y_(n+1)=y_1 (Bourke 1988, Nürnberg 2013)。

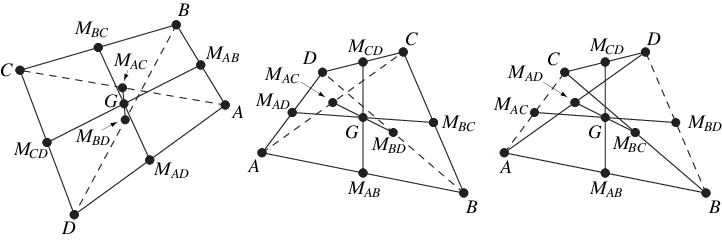
QuadrilateralCentroid

四边形的顶点质心出现在双中线的交点处（即，线 M_(AB)M_(CD) 和 M_(AD)M_(BC) 连接相对中点对）（Honsberger 1995, pp. 36-37）。此外，它也是连接对角线 AC 和 BD 中点的线 M_(AC)M_(BD) 的中点（Honsberger 1995, pp. 39-40）。

给定一个任意六边形，连接每三个连续边的质心会得到所谓的质心六边形，这是一个具有相等且平行边的六边形（Wells 1991）。

半径为 R 的半圆的质心由下式给出

x^_=(2R)/pi.
(10)

下表总结了沿非对称轴的以下曲线所界定的几个常见薄片的质心。

薄片y^_
扇形(4Rsin(1/2theta))/(3theta)
弓形(4Rsin^3(1/2theta))/(3(theta-sintheta))
等腰三角形1/3h
抛物线弓形2/5h
半圆(4R)/(3pi)

在三维中，密度函数为 rho(x,y,z) 的固体的质量是

M=intintintrho(x,y,z)dV,
(11)

质心的坐标是

x^_=(intintintxrho(x,y,z)dV)/M
(12)
y^_=(intintintyrho(x,y,z)dV)/M
(13)
z^_=(intintintzrho(x,y,z)dV)/M.
(14)
固体z^_
圆锥1/4h
圆锥台(h(R_1^2+2R_1R_2+3R_2^2))/(4(R_1^2+R_1R_2+R_2^2))
半椭球体3/(16)a, 3/(16)b, 3/(16)c
半球体3/8R
抛物面体2/3h
棱锥1/4h
球冠(3(2R-h)^2)/(4(3R-h))
拱顶3/8r

另请参阅

质心六边形, 质量外心, 帕普斯质心定理, 多边形质心, 多面体质心

使用 探索

参考文献

Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 132, 1987.Bourke, P. "Calculating the Area and Centroid of a Polygon." July 1988. http://paulbourke.net/geometry/polygonmesh/.Honsberger, R. Episodes in Nineteenth and Twentieth Century Euclidean Geometry. Washington, DC: Math. Assoc. Amer., 1995.Kern, W. F. and Bland, J. R. "Center of Gravity." §39 in Solid Mensuration with Proofs, 2nd ed. New York: Wiley, p. 110, 1948.McLean, W. G. and Nelson, E. W. "First Moments and Centroids." Ch. 9 in Schaum's Outline of Theory and Problems of Engineering Mechanics: Statics and Dynamics, 4th ed. New York: McGraw-Hill, pp. 134-162, 1988.Nürnberg, R. "Calculating the Area and Centroid of a Polygon in 2D." 2013. https://www.ma.imperial.ac.uk/~rn/centroid.pdf.Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, 1999.Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, pp. 53-54, 1991.

在 中被引用

几何质心

请引用本文为

Weisstein, Eric W. "几何质心。" 来自 Web 资源。 https://mathworld.net.cn/GeometricCentroid.html

学科分类