外摆线是由半径为  的圆上一点
的圆上一点  在半径为
在半径为  的圆外部滚动时所描绘的路径。因此,外摆线是 外旋轮线,其中
的圆外部滚动时所描绘的路径。因此,外摆线是 外旋轮线,其中  。外摆线由参数方程给出
。外摆线由参数方程给出
可以通过计算导出极坐标方程
因此
![r^2=x^2+y^2=(a+b)^2+b^2-2b(a+b){cos[(a/b+1)phi]cosphi+sin[(a/b+1)phi]sinphi}.](/images/equations/Epicycloid/NumberedEquation1.svg) |
(5)
|
但是
 |
(6)
|
因此
请注意, 是这里的参数,不是极角。从中心开始的极角是
是这里的参数,不是极角。从中心开始的极角是
 |
(9)
|
为了在外摆线中得到  个尖点,
个尖点, ,因为这样
,因为这样  次
次  的旋转会将边缘上的点带回到其起始位置。
的旋转会将边缘上的点带回到其起始位置。
因此
具有一个尖点的外摆线称为心脏线,具有两个尖点的外摆线称为肾脏线,具有五个尖点的外摆线称为毛茛线。
外摆线也可以通过从圆的直径开始构造,并沿圆周以等弧长的一系列步长偏移一端,同时沿圆周以  倍大的步长偏移另一端。在围绕圆一周后,会产生
倍大的步长偏移另一端。在围绕圆一周后,会产生  尖点外摆线的包络线,如上图所示(Madachy 1979)。
尖点外摆线的包络线,如上图所示(Madachy 1979)。
外摆线具有挠率
 |
(16)
|
并满足
 |
(17)
|
其中  是曲率半径 (
是曲率半径 ( )。
)。
另请参见
心脏线,
环面,
摆线,
外摆线渐屈线,
外摆线渐伸线,
外摆线踏瓣曲线,
外旋轮线,
内摆线,
肾脏线,
毛茛线
使用 探索
参考文献
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 217, 1987.Bogomolny, A. "Cycloids." http://www.cut-the-knot.org/pythagoras/cycloids.shtml.Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 160-164 and 169, 1972.Lemaire, J. Hypocycloïdes et epicycloïdes. Paris: Albert Blanchard, 1967.MacTutor History of Mathematics Archive. "Epicycloid." http://www-groups.dcs.st-and.ac.uk/~history/Curves/Epicycloid.html.Madachy, J. S. Madachy's Mathematical Recreations. New York: Dover, pp. 219-225, 1979.Smith, D. E. History of Mathematics, Vol. 2: Special Topics of Elementary Mathematics. New York: Dover, p. 328, 1958.Wagon, S. Mathematica in Action. New York: W. H. Freeman, pp. 50-52, 1991.Yates, R. C. "Epi- and Hypo-Cycloids." A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 81-85, 1952.
以此引用
Weisstein, Eric W. "外摆线。" 来自 Web 资源。 https://mathworld.net.cn/Epicycloid.html
学科分类
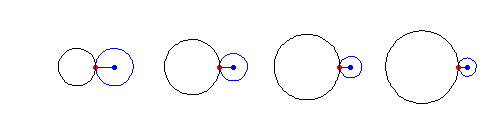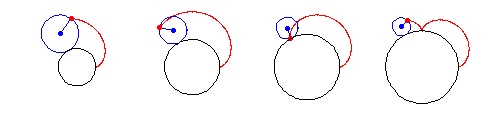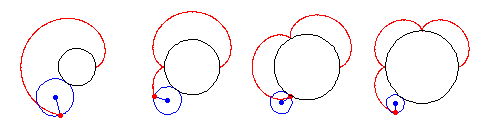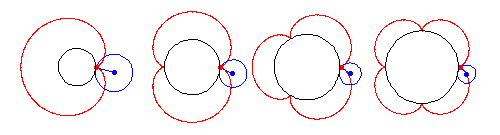
的圆上一点
在半径为
的圆外部滚动时所描绘的路径。因此,外摆线是 外旋轮线,其中
。外摆线由参数方程给出
是这里的参数,不是极角。从中心开始的极角是

个尖点,
,因为这样
次
的旋转会将边缘上的点带回到其起始位置。
![(a((n+1)/n)sinphi-a/nsin[(n+1)phi])/(a((n+1)/n)cosphi-a/ncos[(n+1)phi])](/images/equations/Epicycloid/Inline42.svg)
倍大的步长偏移另一端。在围绕圆一周后,会产生
尖点外摆线的包络线,如上图所示(Madachy 1979)。
是曲率半径 (
)。