狄利克雷eta函数是由  定义的函数,定义如下:
定义的函数,定义如下:
其中  是黎曼zeta函数。请注意,Borwein 和 Borwein (1987, p. 289) 使用符号
是黎曼zeta函数。请注意,Borwein 和 Borwein (1987, p. 289) 使用符号  而不是
而不是  。该函数也称为交错zeta函数,并用
。该函数也称为交错zeta函数,并用  表示 (Sondow 2003, 2005)。
表示 (Sondow 2003, 2005)。
 通过在 (2) 的右侧设置
通过在 (2) 的右侧设置  来定义,而
来定义,而  (有时称为交错调和级数) 是使用左侧定义的。除了
(有时称为交错调和级数) 是使用左侧定义的。除了  之外,该函数在
之外,该函数在  的每个零点处消失 (Sondow 2003)。
的每个零点处消失 (Sondow 2003)。
eta函数与黎曼zeta函数和狄利克雷lambda函数的关系为
 |
(3)
|
和
 |
(4)
|
(Spanier 和 Oldham 1987)。 eta函数也是多对数函数的特例,
 |
(5)
|
值  可以通过注意到
可以通过注意到  对于
对于  的麦克劳林级数为
的麦克劳林级数为
 |
(6)
|
因此,自然对数 2 为
偶数 整数 的值与黎曼zeta函数的解析值有关。特定值在 Abramowitz 和 Stegun (1972, p. 811) 中给出,包括
它出现在积分中
![int_0^1int_0^1([-ln(xy)]^s)/(1+xy)dxdy=Gamma(s+2)eta(s+2)](/images/equations/DirichletEtaFunction/NumberedEquation5.svg) |
(17)
|
(Guillera 和 Sondow 2005)。
eta函数的导数由下式给出
 |
(18)
|
特殊情况由下式给出
(OEIS A271533, OEIS A256358, OEIS A265162, 和 OEIS A091812), 其中  是Glaisher-Kinkelin 常数,
是Glaisher-Kinkelin 常数,  是黎曼zeta函数, 并且
是黎曼zeta函数, 并且  是欧拉-马歇罗尼常数。
是欧拉-马歇罗尼常数。  的恒等式提供了沃利斯公式的一个显著证明。
的恒等式提供了沃利斯公式的一个显著证明。
另请参阅
戴德金Eta函数,
狄利克雷Beta函数,
狄利克雷Lambda函数,
Hadjicostas公式,
黎曼Zeta函数,
Zeta函数
使用 探索
参考文献
Abramowitz, M. 和 Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 807-808, 1972.Borwein, J. M. 和 Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity. New York: Wiley, 1987.Guillera, J. 和 Sondow, J. "Double Integrals and Infinite Products for Some Classical Constants Via Analytic Continuations of Lerch's Transcendent." 2005年6月16日. http://arxiv.org/abs/math.NT/0506319.Havil, J. "Real Alternatives." §16.12 in Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 206-207, 2003.Sloane, N. J. A. 序列 A271533, A256358, A265162, 和 A091812 在 "整数序列在线百科全书" 中.Sondow, J. "Zeros of the Alternating Zeta Function on the Line ![R[s]=1](/images/equations/DirichletEtaFunction/Inline78.svg) ." Amer. Math. Monthly 110, 435-437, 2003.Sondow, J. "Double Integrals for Euler's Constant and
." Amer. Math. Monthly 110, 435-437, 2003.Sondow, J. "Double Integrals for Euler's Constant and  and an Analog of Hadjicostas's Formula." Amer. Math. Monthly 112, 61-65, 2005.Spanier, J. 和 Oldham, K. B. "The Zeta Numbers and Related Functions." Ch. 3 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 25-33, 1987.
and an Analog of Hadjicostas's Formula." Amer. Math. Monthly 112, 61-65, 2005.Spanier, J. 和 Oldham, K. B. "The Zeta Numbers and Related Functions." Ch. 3 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 25-33, 1987.在 中被引用
狄利克雷Eta函数
引用此内容为
Weisstein, Eric W. "狄利克雷Eta函数." 来自 --一个 资源. https://mathworld.net.cn/DirichletEtaFunction.html
学科分类
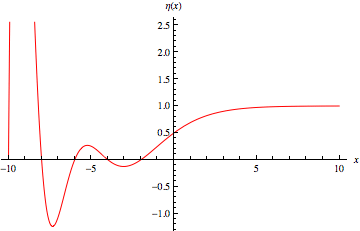
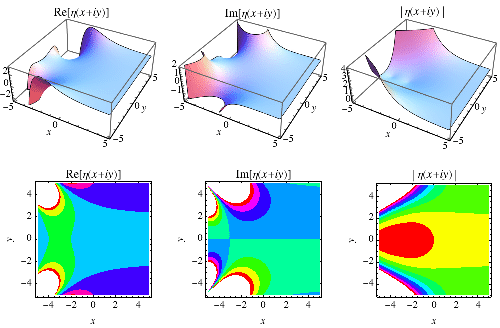
定义的函数,定义如下:

是黎曼zeta函数。请注意,Borwein 和 Borwein (1987, p. 289) 使用符号
而不是
。该函数也称为交错zeta函数,并用
表示 (Sondow 2003, 2005)。
通过在 (2) 的右侧设置
来定义,而
(有时称为交错调和级数) 是使用左侧定义的。除了
之外,该函数在
的每个零点处消失 (Sondow 2003)。
可以通过注意到
对于
的麦克劳林级数为

是Glaisher-Kinkelin 常数,
是黎曼zeta函数, 并且
是欧拉-马歇罗尼常数。
的恒等式提供了沃利斯公式的一个显著证明。