 是由下式定义的三角函数
是由下式定义的三角函数
其中  是余弦。割线在 Wolfram 语言中实现为Sec[z]。
是余弦。割线在 Wolfram 语言中实现为Sec[z]。
请注意,割线函数在欧洲似乎没有得到一致的广泛使用,尽管它确实明确出现在各种德语和俄语手册中(例如,Gradshteyn 和 Ryzhik 2000,第 43 页)。有趣的是,虽然  在某些表格中与其他三角函数并列(Gellert et al. 1989,第 222 页),但在另一些表格中则不然(Gradshteyn 和 Ryzhik 2000,他们在第 28 页的基本函数关系表中没有列出它,但在第 43 页给出了涉及它的恒等式)。
在某些表格中与其他三角函数并列(Gellert et al. 1989,第 222 页),但在另一些表格中则不然(Gradshteyn 和 Ryzhik 2000,他们在第 28 页的基本函数关系表中没有列出它,但在第 43 页给出了涉及它的恒等式)。
Tropfke 指出,“割线函数的历史几乎与正切函数的历史同时开始,但在 17 世纪上半叶对数计算发现后结束”(Tropfke 1923,第 28 页)并且,“当对数引入后,分母中三角函数的出现不再构成任何困难时,割线自然地再次从三角学中消失”(Tropfke 1923,第 30 页)。Harris 和 Stocker(1998,第 300 页)称割线和余割是“极少使用的函数”,但随后用整整一节来专门介绍它们。因为这些函数确实似乎在美国得到广泛使用(例如,Abramowitz 和 Stegun 1972,第 72 页),所以关于它们消亡的报道似乎有点为时过早。
导数是
 |
(3)
|
和不定积分是
![intseczdz=ln[cos(1/2z)+sin(1/2z)]-ln[cos(1/2z)-sin(1/2z)]+C,](/images/equations/Secant/NumberedEquation2.svg) |
(4)
|
其中  是积分常数。对于
是积分常数。对于  ,这可以写成
,这可以写成
割线的麦克劳林级数是
(OEIS A046976 和 A046977),其中  是欧拉数。前几个既约分子是素数的分子为 5, 61, 277, 23489580527043108252017828576198947741, ... (OEIS A092838),对应于
是欧拉数。前几个既约分子是素数的分子为 5, 61, 277, 23489580527043108252017828576198947741, ... (OEIS A092838),对应于  , 3, 4, 19, 24, ... (OEIS A092837)。
, 3, 4, 19, 24, ... (OEIS A092837)。
上面说明了  的分岔图(Trott 2004,第 169 页)。在所有三角函数中,
的分岔图(Trott 2004,第 169 页)。在所有三角函数中, 显然是唯一一个为此形式的迭代显示有趣分岔结构的函数。
显然是唯一一个为此形式的迭代显示有趣分岔结构的函数。
给出  的正整数值,这些值给出
的正整数值,这些值给出  的增量最大值,由 1, 2, 5, 8, 11, 344, 699, 1054, 1409, 1764, 2119, ... (OEIS A004112) 给出,对应于值 1.85082, 2.403, 3.52532, 6.87285, 225.953, 227.503, ....
的增量最大值,由 1, 2, 5, 8, 11, 344, 699, 1054, 1409, 1764, 2119, ... (OEIS A004112) 给出,对应于值 1.85082, 2.403, 3.52532, 6.87285, 225.953, 227.503, ....
另请参阅
交错排列,
余割,
余弦,
欧拉数,
外割线,
双曲割线,
反割线,
割线
相关 Wolfram 网站
http://functions.wolfram.com/ElementaryFunctions/Sec/
使用 探索
参考文献
Abramowitz, M. and Stegun, I. A. (Eds.). "Circular Functions." §4.3 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 71-79, 1972.Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 224, 1987.Gellert, W.; Gottwald, S.; Hellwich, M.; Kästner, H.; and Künstner, H. (Eds.). VNR Concise Encyclopedia of Mathematics, 2nd ed. New York: Van Nostrand Reinhold, 1989.Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, 2000.Harris, J. W. and Stocker, H. "Secant and Cosecant." §5.34 in Handbook of Mathematics and Computational Science. New York: Springer-Verlag, pp. 300-307, 1998.Jeffrey, A. "Trigonometric Identities." §2.4 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 111-117, 2000.Sloane, N. J. A. Sequences A004112, A046976, A046977, A092837, and A092838 in "The On-Line Encyclopedia of Integer Sequences."Spanier, J. and Oldham, K. B. "The Secant  and Cosecant
and Cosecant  Functions." Ch. 33 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 311-318, 1987.Tropfke, J. Teil IB, §3. "Die Begriffe von Sekans und Kosekans eines Winkels." In Geschichte der Elementar-Mathematik in systematischer Darstellung mit besonderer Berücksichtigung der Fachwörter, fünfter Band, zweite aufl. Berlin and Leipzig, Germany: de Gruyter, pp. 28-30, 1923.Trott, M. The Mathematica GuideBook for Programming. New York: Springer-Verlag, 2004. http://www.mathematicaguidebooks.org/.Zwillinger, D. (Ed.). "Trigonometric or Circular Functions." §6.1 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 452-460, 1995.
Functions." Ch. 33 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 311-318, 1987.Tropfke, J. Teil IB, §3. "Die Begriffe von Sekans und Kosekans eines Winkels." In Geschichte der Elementar-Mathematik in systematischer Darstellung mit besonderer Berücksichtigung der Fachwörter, fünfter Band, zweite aufl. Berlin and Leipzig, Germany: de Gruyter, pp. 28-30, 1923.Trott, M. The Mathematica GuideBook for Programming. New York: Springer-Verlag, 2004. http://www.mathematicaguidebooks.org/.Zwillinger, D. (Ed.). "Trigonometric or Circular Functions." §6.1 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 452-460, 1995.在 上被引用
割线
请引用为
Weisstein, Eric W. “割线。” 来自 Web 资源。 https://mathworld.net.cn/Secant.html
学科分类
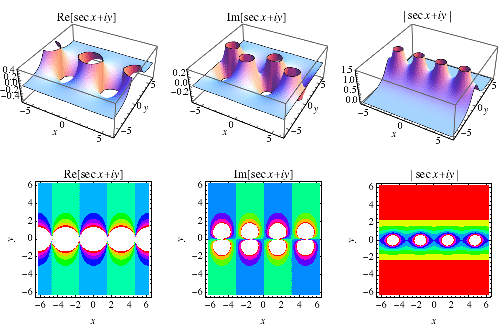
是由下式定义的三角函数
是余弦。割线在 Wolfram 语言中实现为Sec[z]。
在某些表格中与其他三角函数并列(Gellert et al. 1989,第 222 页),但在另一些表格中则不然(Gradshteyn 和 Ryzhik 2000,他们在第 28 页的基本函数关系表中没有列出它,但在第 43 页给出了涉及它的恒等式)。
是积分常数。对于
,这可以写成
是欧拉数。前几个既约分子是素数的分子为 5, 61, 277, 23489580527043108252017828576198947741, ... (OEIS A092838),对应于
, 3, 4, 19, 24, ... (OEIS A092837)。
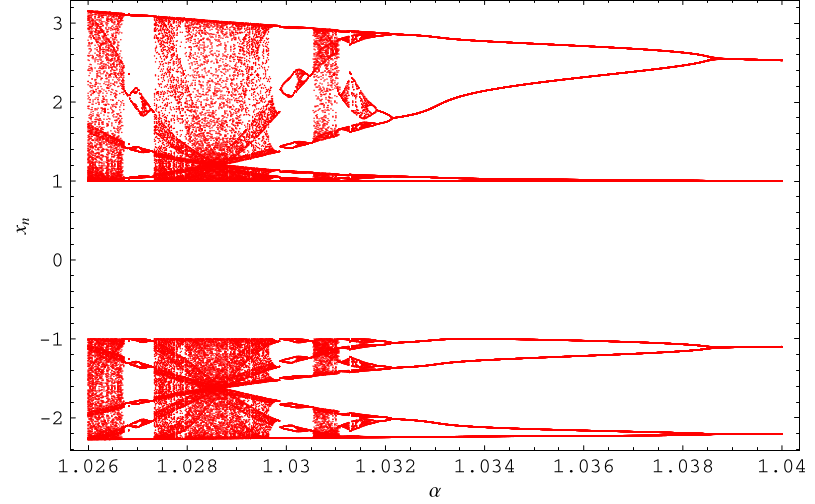
的分岔图(Trott 2004,第 169 页)。在所有三角函数中,
显然是唯一一个为此形式的迭代显示有趣分岔结构的函数。
的正整数值,这些值给出
的增量最大值,由 1, 2, 5, 8, 11, 344, 699, 1054, 1409, 1764, 2119, ... (OEIS A004112) 给出,对应于值 1.85082, 2.403, 3.52532, 6.87285, 225.953, 227.503, ....