古德曼函数

最小值 最大值

古德曼函数是一个奇函数，用 或 表示，它出现在墨卡托投影的反方程中。 表示纬度 用垂直位置 在此投影中表示，因此古德曼函数定义为

对于实数 ，此定义也等于

古德曼函数在 Wolfram 语言 中实现为古德曼函数[z].

古德曼函数的导数为

其不定积分为

其中 是双对数函数。

它具有麦克劳林级数

(OEIS A091912 和 A136606)。

古德曼函数通过以下方式连接了三角函数和双曲函数

古德曼函数通过以下方式与指数函数相关

(Beyer 1987, p. 164; Zwillinger 1995, p. 485)。

其他基本恒等式为

(Zwillinger 1995, p. 485)。

如果 ，那么

(Beyer 1987, p. 164; Zwillinger 1995, p. 530)，其中最后一个恒等式已被更正。

另一个恒等式由下式给出

(M. Somos, 私人通信，2006 年 4 月 15 日)。

最小值 最大值 实部 虚部

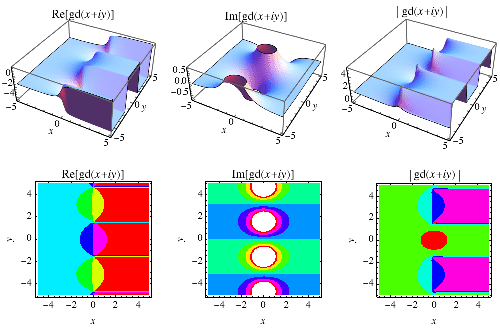古德曼函数也可以扩展到复平面，如上图所示。