Pochhammer 符号
(Abramowitz 和 Stegun 1972, p. 256; Spanier 1987; Koepf 1998, p. 5) 对于  是一种不幸的记号,在特殊函数理论中用于 上升阶乘,也称为上升阶乘幂 (Graham et al. 1994, p. 48) 或上升阶乘 (Boros 和 Moll 2004, p. 16)。Pochhammer 符号在 Wolfram 语言 中实现为Pochhammer[x, n].
是一种不幸的记号,在特殊函数理论中用于 上升阶乘,也称为上升阶乘幂 (Graham et al. 1994, p. 48) 或上升阶乘 (Boros 和 Moll 2004, p. 16)。Pochhammer 符号在 Wolfram 语言 中实现为Pochhammer[x, n].
在组合数学中,记号  (Roman 1984, p. 5)、
(Roman 1984, p. 5)、 (Comtet 1974, p. 6) 或
(Comtet 1974, p. 6) 或  (Graham et al. 1994, p. 48) 用于 上升阶乘,而
(Graham et al. 1994, p. 48) 用于 上升阶乘,而  或
或  表示 下降阶乘 (Graham et al. 1994, p. 48)。因此,在解释记号
表示 下降阶乘 (Graham et al. 1994, p. 48)。因此,在解释记号  和
和  时需要格外小心。
时需要格外小心。
 对于非负整数
对于非负整数  的前几个值为
的前几个值为
(OEIS A054654)。
以闭合形式, 可以写成
可以写成
 |
(8)
|
其中  是 第一类斯特林数。
是 第一类斯特林数。
Pochhammer 符号满足
 |
(9)
|
二等分公式
和倍增公式
 |
(12)
|
(Boros 和 Moll 2004, p. 17)。
Pochhammer 符号的比率以闭合形式给出为
 |
(13)
|
(Boros 和 Moll 2004, p. 17)。
导数由下式给出
![d/(dx)(x)_n=(x)_n[psi_0(n+x)-psi_0(x)],](/images/equations/PochhammerSymbol/NumberedEquation5.svg) |
(14)
|
其中  是 双伽玛函数。
是 双伽玛函数。
特殊值包括
Pochhammer 符号  服从欧拉的变换
服从欧拉的变换
 |
(17)
|
其中  是 前向差分,并且
是 前向差分,并且
 |
(18)
|
(Nørlund 1955)。
 的和可以以闭合形式完成,如下所示
的和可以以闭合形式完成,如下所示
 |
(19)
|
对于  。
。
考虑乘积
此函数收敛到 0、有限值或发散,具体取决于  的值。临界曲线由 隐式方程 给出
的值。临界曲线由 隐式方程 给出
![R[-1+ln(z^(-z)(1+z)^(1+z))]=0.](/images/equations/PochhammerSymbol/NumberedEquation9.svg) |
(22)
|
在此曲线内部,函数收敛到 0,而在其外部,函数发散。发生收敛的最大实数值由  (OEIS A090462) 给出,最小值由
(OEIS A090462) 给出,最小值由  给出。
给出。 的极值由
的极值由  (OEIS A090463) 给出。在临界轮廓上,
(OEIS A090463) 给出。在临界轮廓上, 取值为
取值为
![f(z)=1/2[lnz+ln(z+1)].](/images/equations/PochhammerSymbol/NumberedEquation10.svg) |
(23)
|
绘制适当缩放版本的  ,其中
,其中  是有限的,显示出美丽而微妙的结构,例如上面为
是有限的,显示出美丽而微妙的结构,例如上面为  所示的结构 (M. Trott,私人通讯,2003 年 12 月 1 日)。
所示的结构 (M. Trott,私人通讯,2003 年 12 月 1 日)。
另一个精美的可视化图绘制了  ,如上面为
,如上面为  所示 (M. Trott,私人通讯,2003 年 12 月 2 日)。
所示 (M. Trott,私人通讯,2003 年 12 月 2 日)。
另请参阅
阶乘,
下降阶乘,
广义超几何函数,
Hankel 符号,
调和对数,
超几何函数,
Kramp 符号,
上升阶乘
相关的 Wolfram 网站
http://functions.wolfram.com/GammaBetaErf/Pochhammer/
使用 探索
参考文献
Abramowitz, M. 和 Stegun, I. A. (编). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, 1972.Boros, G. 和 Moll, V. Irresistible Integrals: Symbolics, Analysis and Experiments in the Evaluation of Integrals. Cambridge, England: Cambridge University Press, 2004.Comtet, L. Advanced Combinatorics: The Art of Finite and Infinite Expansions, 修订增补版. Dordrecht, Netherlands: Reidel, 1974.Erdélyi, A.; Magnus, W.; Oberhettinger, F.; 和 Tricomi, F. G. Higher Transcendental Functions, Vol. 1. New York: Krieger, p. 52, 1981.Graham, R. L.; Knuth, D. E.; 和 Patashnik, O. Concrete Mathematics: A Foundation for Computer Science, 2nd ed. Reading, MA: Addison-Wesley, 1994.Koepf, W. Hypergeometric Summation: An Algorithmic Approach to Summation and Special Function Identities. Braunschweig, Germany: Vieweg, 1998.Nørlund, N. E. "Hypergeometric Functions." Acta Math. 94, 289-349, 1955.Roman, S. The Umbral Calculus. New York: Academic Press, p. 5, 1984.Sloane, N. J. A. Sequences A054654, A090462, and A090463 in "The On-Line Encyclopedia of Integer Sequences."Spanier, J. 和 Oldham, K. B. "The Pochhammer Polynomials  ." 第 18 章,载于 An Atlas of Functions. Washington, DC: Hemisphere, pp. 149-165, 1987.
." 第 18 章,载于 An Atlas of Functions. Washington, DC: Hemisphere, pp. 149-165, 1987.在 中被引用
Pochhammer 符号
请引用为
Weisstein, Eric W. "Pochhammer 符号。" 来自 Web 资源。 https://mathworld.net.cn/PochhammerSymbol.html
主题分类
是一种不幸的记号,在特殊函数理论中用于 上升阶乘,也称为上升阶乘幂 (Graham et al. 1994, p. 48) 或上升阶乘 (Boros 和 Moll 2004, p. 16)。Pochhammer 符号在 Wolfram 语言 中实现为Pochhammer[x, n].
(Roman 1984, p. 5)、
(Comtet 1974, p. 6) 或
(Graham et al. 1994, p. 48) 用于 上升阶乘,而
或
表示 下降阶乘 (Graham et al. 1994, p. 48)。因此,在解释记号
和
时需要格外小心。
对于非负整数
的前几个值为
可以写成

是 第一类斯特林数。


是 双伽玛函数。
服从欧拉的变换
是 前向差分,并且

的和可以以闭合形式完成,如下所示

。
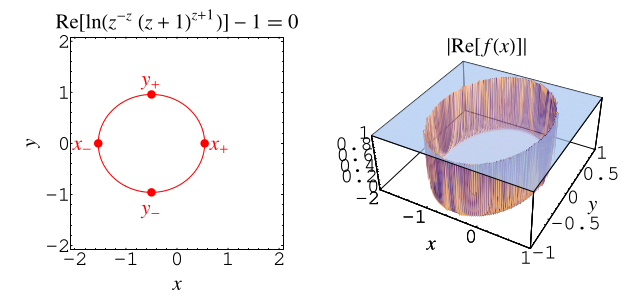

的值。临界曲线由 隐式方程 给出
(OEIS A090462) 给出,最小值由
给出。
的极值由
(OEIS A090463) 给出。在临界轮廓上,
取值为
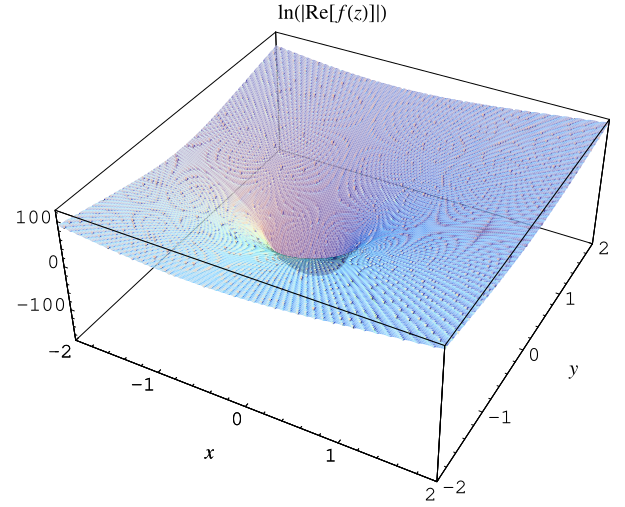
,其中
是有限的,显示出美丽而微妙的结构,例如上面为
所示的结构 (M. Trott,私人通讯,2003 年 12 月 1 日)。
,如上面为
所示 (M. Trott,私人通讯,2003 年 12 月 2 日)。