主题
Search

正弦积分

下载 Mathematica 笔记本下载 Wolfram 笔记本
SinIntegralReal
最小值 最大值
Powered by webMathematica
SinIntegralReImAbs
最小值 最大值
实部
虚部 Powered by webMathematica

最常见的“正弦积分”定义为

Si(z)=int_0^z(sint)/tdt
(1)

Si(z) 是在 Wolfram 语言 中实现的函数，函数名为SinIntegral[z]。

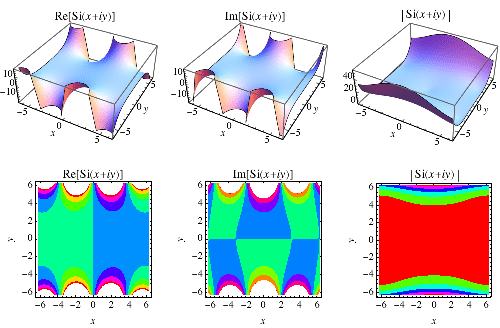
Si(z) 是一个 整函数。

一个密切相关的函数定义为

si(x)=-int_x^infty(sint)/tdt
(2)
=1/(2i)[Ei(ix)-Ei(-ix)]
(3)
=1/(2i)[e_1(ix)-e_1(-ix)]
(4)
=Si(z)-1/2pi,
(5)

其中 Ei(x) 是 指数积分，(3) 对 x<0 成立，并且

e_1(x)=-Ei(-x).
(6)

导数 Si(x) 是

d/(dx)Si(x)=sinc(x),
(7)

其中 sinc(x) 是 sinc 函数，积分 是

intSi(x)dx=cosx+xSi(x).
(8)

对于 Si(x) 的级数由下式给出

Si(x)=sum_(k=1)^infty(-1)^(k-1)(x^(2k-1))/((2k-1)(2k-1)!)
(9)

(Havil 2003, p. 106)。

它可以用 第一类球贝塞尔函数 展开，形式为

Si(2x)=2xsum_(n=0)^infty[j_n(x)]^2
(10)

(Harris 2000)。

sinc 函数的半无限积分由下式给出

si(0)=-int_0^infty(sinx)/xdx=-1/2pi.
(11)

要计算正弦函数乘以幂的积分

I=intx^(2n)sin(mx)dx,
(12)

使用 分部积分法。设

u=x^(2n) dv=sin(mx)dx
(13)
du=2nx^(2n-1)dx v=-1/mcos(mx),
(14)

所以

I=-1/mx^(2n)cos(mx)+(2n)/mintx^(2n-1)cos(mx)dx.
(15)

再次使用 分部积分法，

u=x^(2n-1) dv=cos(mx)dx
(16)
du=(2n-1)x^(2n-2)dx v=1/msin(mx)
(17)
intx^(2n)sin(mx)dx=-1/mx^(2n)cos(mx) +(2n)/m[1/mx^(2n-1)cos(mx)-(2n-1)/mintx^(2n-2)sin(mx)dx] =-1/mx^(2n)sin(mx)+(2n)/(m^2)x^(2n-1)sin(mx)-((2n)(2n-1))/(m^2)intx^(2n-2)sin(mx)dx =-1/mx^(2n)cos(mx)+(2n)/(m^2)x^(2n-1)sin(mx)+...+((2n)!)/(m^(2n))intx^0sin(mx)dx =-1/mx^(2n)cos(mx)+(2n)/(m^2)x^(2n-1)sin(mx)+...-((2n)!)/(m^(2n+1))cos(mx) =cos(mx)sum_(k=0)^n(-1)^(k+1)((2n)!)/((2n-2k)!m^(2k+1))x^(2n-2k) +sin(mx)sum_(k=1)^n(-1)^(k+1)((2n)!)/((2k-2n-1)!m^(2k))x^(2n-2k+1).
(18)

令 k^'=n-k，所以

intx^(2n)sin(mx)dx =cos(mx)sum_(k=0)^n(-1)^(n-k+1)((2n)!)/((2k)!m^(2n-2k+1))x^(2k)+sin(mx)sum_(k=0)^(n-1)(-1)^(n-k+1)((2n)!)/((2k-1)!m^(2n-2k))x^(2k+1) =(-1)^(n+1)(2n)![cos(mx)sum_(k=0)^n((-1)^k)/((2k)!m^(2n-2k+1))x^(2k)+sin(mx)sum_(k=1)^n((-1)^(k+1))/((2k-3)!m^(2n-2k+2))x^(2k-1)].
(19)

形式为的一般积分

I(k,l)=int_0^infty(sin^kx)/(x^l)dx
(20)

与 sinc 函数 相关，并且可以解析计算。

另请参阅

Chi, 余弦积分, 指数积分, 尼尔森螺线, Shi, Sinc 函数

相关的 Wolfram 网站

http://functions.wolfram.com/GammaBetaErf/SinIntegral/

使用 探索

参考文献

Abramowitz, M. 和 Stegun, I. A. (编辑). "Sine and Cosine Integrals." §5.2 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 231-233, 1972.Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 342-343, 1985.Harris, F. E. "Spherical Bessel Expansions of Sine, Cosine, and Exponential Integrals." Appl. Numer. Math. 34, 95-98, 2000.Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 105-106, 2003.Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; 和 Vetterling, W. T. "Fresnel Integrals, Cosine and Sine Integrals." §6.79 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 248-252, 1992.Spanier, J. 和 Oldham, K. B. "The Cosine and Sine Integrals." Ch. 38 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 361-372, 1987.

在 中引用

正弦积分

请引用为

Weisstein, Eric W. “正弦积分。” 来自 —— 资源。 https://mathworld.net.cn/SineIntegral.html

主题分类