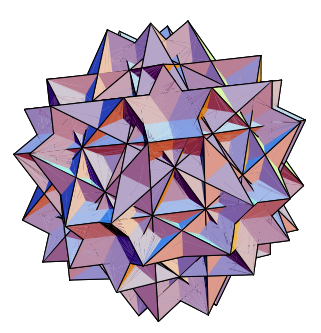
拟菱形二十-十二面体

拟菱形二十-十二面体是均匀多面体，Maeder 索引为 67 (Maeder 1997)，Wenninger 索引为 105 (Wenninger 1989)，Coxeter 索引为 84 (Coxeter 等人 1954)，Har'El 索引为 72 (Har'El 1993)。它的施莱夫利符号为 r'，Wythoff 符号为。它的面为。

不幸的是，一些作者（例如，Maeder 1997，模型 67）使用术语“大菱形二十-十二面体”来指代这个立体，尽管事实上相同的术语通常被使用（例如，Cundy 和 Rowlett 1989，第 112-113 页）来指代一个不同的阿基米德立体。

拟菱形二十-十二面体在 Wolfram 语言中实现为UniformPolyhedron[105], UniformPolyhedron["GreatRhombicosidodecahedron"], UniformPolyhedron["Coxeter", 84], UniformPolyhedron["Kaleido", 72], UniformPolyhedron["Uniform", 67], 或UniformPolyhedron["Wenninger", 105]. 它也在 Wolfram 语言中实现为PolyhedronData["Quasirhombicosidodecahedron"].

它的骨架是小菱形二十-十二面体图，如上图在几个嵌入中所示。

它的对偶是大三角六十面体。

对于单位边长，它的外接球半径为

另请参阅

菱形二十-十二面体, 均匀多面体

使用探索

更多尝试

参考文献

Coxeter, H. S. M.; Longuet-Higgins, M. S.; and Miller, J. C. P. "Uniform Polyhedra." Phil. Trans. Roy. Soc. London Ser. A 246, 401-450, 1954.Cundy, H. and Rollett, A. "Great Rhombicosidodecahedron or Truncated Icosidodecahedron.

." §3.7.12 在 Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., 页 112-113, 1989.Har'El, Z. "Uniform Solution for Uniform Polyhedra." Geometriae Dedicata 47, 57-110, 1993.Maeder, R. E. "67: Great Rhomibicosidodecahedron." 1997. https://www.mathconsult.ch/static/unipoly/67.html.Wenninger, M. J. "Quasirhombicosidecahedron." 模型 105 在 Polyhedron Models. Cambridge, England: Cambridge University Press, 页 162-163, 1989.

请引用本文为

Weisstein, Eric W. “拟菱形二十-十二面体。” 来自 Web 资源。 https://mathworld.net.cn/Quasirhombicosidodecahedron.html