魔鬼曲线由 G. Cramer 于 1750 年和 Lacroix 于 1810 年研究 (MacTutor Archive)。它于 1858 年出现在 Nouvelles Annales 中。笛卡尔方程为
 |
(1)
|
等价于
 |
(2)
|
极坐标方程为
 |
(3)
|
参数方程为
上面所示的曲线对应于参数  和
和  。
。
它在原点有一个叉点。
对于  ,中心的沙漏是水平的,对于
,中心的沙漏是水平的,对于  ,它是垂直的,并且当它经过
,它是垂直的,并且当它经过  时,曲线变为一个圆。
时,曲线变为一个圆。
魔鬼曲线的一个特例是所谓的“电动马达曲线”
 |
(6)
|
(Cundy 和 Rollett 1989)。
另请参阅
哑铃图,
蝴蝶曲线,
哑铃曲线,
八字曲线,
双纽线,
梨形曲线,
叉形分岔,
泪珠曲线
使用 探索
参考文献
--. Nouvelle Annales, p. 317, 1858.Cramer, G. Introduction a l'analyse des lignes courbes algébriques. Geneva, p. 19, 1750.Cundy, H. and Rollett, A. Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., p. 71, 1989.Gray, A. Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, pp. 92-93, 1997.Lacroix, S. F. Traité du calcul différentiel et intégral, Vol. 1. Paris, p. 391, 1810.Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 151-152, 1972.MacTutor History of Mathematics Archive. "Devil's Curve." http://www-groups.dcs.st-and.ac.uk/~history/Curves/Devils.html.Smith, D. E. History of Mathematics, Vol. 2: Special Topics of Elementary Mathematics. New York: Dover, p. 328, 1958.
请引用为
Weisstein, Eric W. "Devil's Curve." 来自 ——Wolfram 网络资源。 https://mathworld.net.cn/DevilsCurve.html
学科分类


和
。
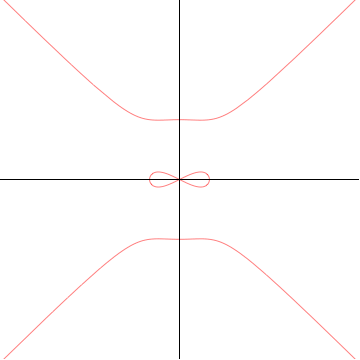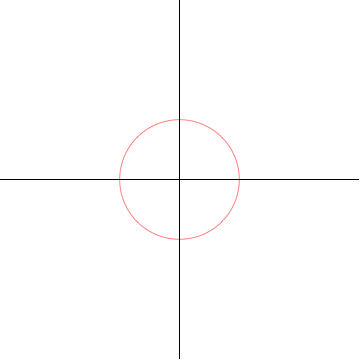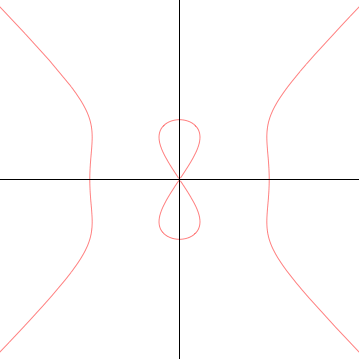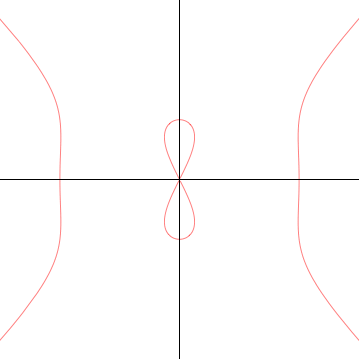
,中心的沙漏是水平的,对于
,它是垂直的,并且当它经过
时,曲线变为一个圆。