由下式定义的一般四次曲面
 |
(1)
|
(Gray 1997, 第 314 页)。 上面两幅图像分别对应于  , 和
, 和  , 分别地。
, 分别地。
上面展示了其他情况。
对应于  的“圆角立方体”情况是体积为 超椭球体
的“圆角立方体”情况是体积为 超椭球体
 |
(2)
|
其中  是 伽玛函数。
是 伽玛函数。
情况  的体积由下式给出
的体积由下式给出
其中
 |
(5)
|
![R[z]](/images/equations/GoursatsSurface/Inline12.svg) 是
是  的实部,而
的实部,而  是第一类贝塞尔函数 (E. W. Weisstein 和 M. Trott,私人通讯,2008 年 11 月 9 日),这可能可以用二元超几何函数以闭合形式表示。
是第一类贝塞尔函数 (E. W. Weisstein 和 M. Trott,私人通讯,2008 年 11 月 9 日),这可能可以用二元超几何函数以闭合形式表示。
相关的曲面
 |
(6)
|
对于  的偶数整数,Gray(1997,第 292 页)也考虑了这种情况,它是超椭球体的一个特例。
的偶数整数,Gray(1997,第 292 页)也考虑了这种情况,它是超椭球体的一个特例。
另请参阅
Chmutov 曲面,
立方体,
超椭球体,
齿状曲面
使用 探索
参考文献
Banchoff, T. F. "Computer Graphics Tools for Rendering Algebraic Surfaces and for Geometry of Order." In Geometric Analysis and Computer Graphics: Proceedings of a Workshop Held May 23-25, 1988 (Eds. P. Concus, R. Finn, D. A. Hoffman). New York: Springer-Verlag, pp. 31-37, 1991.Goursat, E. "Étude des surfaces qui admettent tous les plans de symétrie d'un polyèdre régulier." Ann. Sci. École Norm. Sup. 4, 159-2000, 1897.Gray, A. Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, pp. 292 and 314, 1997.
请引用为
Weisstein, Eric W. “Goursat 曲面。” 来自 Web 资源。 https://mathworld.net.cn/GoursatsSurface.html
主题分类
, 和
, 分别地。
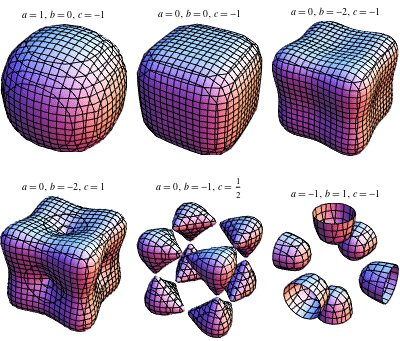
的“圆角立方体”情况是体积为 超椭球体

是 伽玛函数。
的体积由下式给出

![int_0^infty-(pi^2)/(16sqrt(2))R{((-1)^(5/8))/(t^(7/4))[(-1)^(1/4)e^(it/8)t^(3/4)×[(-1)^(3/4)J_(1/4)(t/8)-J_(-1/4)(t/8)]^3+(12sqrt(t)Gamma(1/4))/(pi^2)+(2(1+i)Gamma^3(1/4))/(pi^3)]}dt](/images/equations/GoursatsSurface/Inline11.svg)
是
的实部,而
是第一类贝塞尔函数 (E. W. Weisstein 和 M. Trott,私人通讯,2008 年 11 月 9 日),这可能可以用二元超几何函数以闭合形式表示。
的偶数整数,Gray(1997,第 292 页)也考虑了这种情况,它是超椭球体的一个特例。