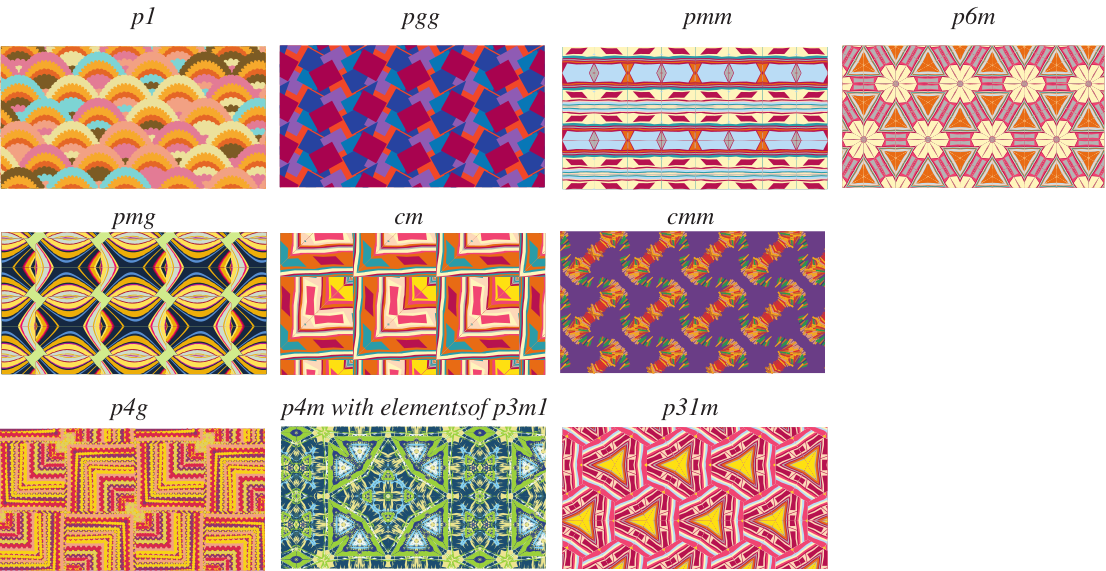
壁纸群是17种可能的平面对称群。它们通常使用类Hermann-Mauguin符号或 orbifold 符号表示 (Zwillinger 1995, p. 260)。
上面展示了使用 Artlandia SymmetryWorks 为每个群组创建的图案。
通过根据壁纸群的对称性重复几何和艺术图案，可以创建美丽的图案，正如 M. C. Escher 的作品以及 I. Bakshee 在 Wolfram 语言 中使用 Artlandia 创建的图案所例证，如上图所示。
有关每个空间群中存在的对称元素的描述，请参阅 Coxeter (1969, p. 413)。
另请参阅
晶体学点群,
空间群,
镶嵌,
平铺
使用 Wolfram|Alpha 探索
参考文献
Artlandia. "Artlandia: Practical, Easy-to-Use Tools for Graphic Design." http://www.artlandia.com/.Bakshee, I. Graphica 2: The World of Mathematica Graphics. The Beauty of Random Worlds: The Art of Igor Bakshee. Wellesley, MA: A K Peters, 1999.Baloglou, G. "Crystallography Now." http://www.oswego.edu/~baloglou/103/seventeen.html.Coxeter, H. S. M. Introduction to Geometry, 2nd ed. New York: Wiley, 1969.Escher, M. C. "Picture Gallery 'Symmetry.' " http://www.mcescher.com/Gallery/gallery-symmetry.htm.Hilbert, D. and Cohn-Vossen, S. Geometry and the Imagination. New York: Chelsea, 1999.Joyce, D. E. "Wallpaper Groups (Plane Symmetry Groups)." http://aleph0.clarku.edu/~djoyce/wallpaper/.Schattschneider, D. "The Plane Symmetry Groups: Their Recognition and Notation." Amer. Math. Monthly 85, 439-450, 1978.Weyl, H. Symmetry. Princeton, NJ: Princeton University Press, 1952.Zwillinger, D. (Ed.). "Crystallographic Groups." §4.2.4 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 259-264, 1995.在 Wolfram|Alpha 中被引用
壁纸群
请引用为
Weisstein, Eric W. "壁纸群。" 来自 MathWorld——Wolfram Web 资源。 https://mathworld.net.cn/WallpaperGroups.html
主题分类
